The Cave Rings Mystery
Le Mystère des Cercles Noirs
Some very special speleothems
The perfect circles drawn on the floor of some caves are mysterious speleothems. Usually rare, they can be abundant in some places. We had the opportunity to meet such forms several times during our expeditions in Laos. The first time in 2004, in Tham Mo (also named Tham Pha Leusi, Ban Vang Hin, upper Nam Pakan valley), then in 2007, in Tham En (Ban Nong Ping, Xe Bang Fai valley), and finally in 2008 in the Cave of the Clouds (Xe Bang Fai cave).
Photo 1 and 1a - Black cave ring in Tham En, Laos ( R. Huttler, 2008)
However, these very special speleothems are not new. F. Brouquisse has reported similar observations in Tham Boumlou (lower Nam Pakan river cave - Gallery of the Stone Sentinels) in 2002. In North Laos, near Van Vieng, Renouard et al. (2001) have observed hundreds of these rings in Tham Lom. They are not exclusive to Laos and some observations, made in Brazil and Italy, have already been published (Montanaro, 1992; Auler, 1993; Hill and Forti, 1998 ...). The phenomenon seemed interesting and unusual enough to trigger, in 2008, a thorough study of some of its aspects.
The rings occur most often as dark traces, usually sharp as long as the diameter is not significant (less than one meter). The center of the rings is usually occupied by a stalagmite which height does not exceed usualy ten centimeters. The morphology of the stalagmite shows no distinctive feature. The traces correspond to a one or two centimeter wide and one millimeter thick deposit in the case of the best drawn rings. This deposit can sometimes be detached (crust) and some micro-samples have been taken for analytical purposes. The rings are more diffuse when their diameter increases. The largest are 5 m in diameter and appear as a faint trace.
The ground nature does not matter and the rings can be present on boulders or on soils of calcite or clay. In the latter case, the rings have been observed in areas flooded and covered with clay during the rainy season. This indicates that the rings can be formed within a few months, when the pools are dry, between two rain seasons. A sampling across the clay deposits would bring interesting informations.
In Tham En, in February 2008, the diameters and ceiling heights of about forty rings were measured in different area of the cave. The diameter measurement is usually not a problem, except when the ground is sloping. Then the rings adopt an elliptical shape whith a major axis oriented in the direction of steepest descent. In this case, we retained as characteristic dimension the length of the ellipse minor axis passing through the central stalagmite. The ceiling height was more difficult to assess. A laser distance meter has a sufficient accuracy provided of course that the target can be defined with certainty. This was the main source of difficulty because, in the absence of reference to define accurately the vertical, it was not possible to spot the starting point of the drops fall. When the passage height exceeded 15 meters, we could barely distinguish the ceiling in the light of our lamps. We then proceeded through trial and error, making series of measurements, and assuming the lowest height found was that of the original stalactite. The results are presented on Fig. 1. The data align on a straight line with a small offset.
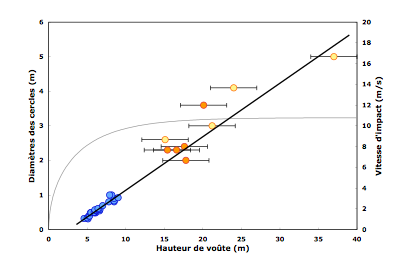
Figure 1 - Relationship between passage height and ring diameter in Tham En. The color of the dots corresponds to three series of measurements in different areas of the cave. The impact velocity (thin line), calculated for a 5 mm diameter water droplet is also plotted on the graph.
Two mechanisms have been proposed to explain these formations. Hill and Forti (1998) believed that the ring formation was related to the droplets projection after impact on th
e ground of drops falling from the roof (splash rings). However, it seems very unlikely that a mere rebound could be the ring formation mechanism. This would require the drops to bounce and be split regardless of the nature and geometry of the ground, then to settle as perfect circles measuring sometimes several meters in diameter. The photo 2 shows that this is not what is usually observed ! Even if this might be possible in a limited number of occasions, the fact that the rings are often grouped on a limited area requires to re-assert the problem. Additionnaly, the rings diameter should be directly related to the drops velocity at impact on the ground. This velocity increases first as a function of the fall height according to the equation v2 = 2 gh, but then reaches progressively a constant value due to the friction with air (drag force).
The impact speed of 5 mm diameter water drops, calculated taking into account the air friction, has been reported on Figure 1. There is no correlation between the evolution of drop velocity and ring diameter. From 15 to 30 m fall height, the impact speed do not change much, while the ring diameters increase from 2 to 5 meters. Such decisive observations could be made only in a large cave. Tham En, where the passage size locally exceeds 100 x 100 m, has been a privileged site of observation.
Photo 2 - Random and asymetric trajectories of splash droplets after ground impact
Finally, several observations show that the rings can be formed even when the impact point on the ground takes place in a crevice preventing lateral projections. This is clearly demonstrated with the ring deposited on a broken block, found in the Cave of the Clouds: seen from above, it has the appearance of a "normal" ring, albeit discontinuous, while the drop impact point is about twenty centimeters below, between the blocks. Seen from the side, the circle is developed on several levels.
Photo 3 and 3 bis - The broken ring in the Cave of the Clouds, top and side view.
The impact point is in between the broken rock . The shuunto is lying on the upper piece.
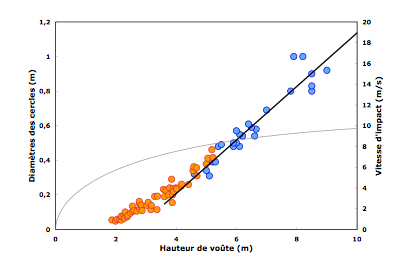
Figure 2 - Correlation between ring diameter and ceiling height in Tham En. The impact velocity, calculated for a 5 mm drop, is also reported.
A remarkable point is the excellent agreement between measurements made by Nozzoli et al. and those conducted by our team in Tham En (Fig. 2). While Nozzoli et al. data and our data cover a different rings size range, the relationship between diameter and ceiling height is remarkably continuous. The quadratic relationship that Nozzoli et al. thought they had highlighted, extends as a straight line. Moreover, mathematical modeling of Montanaro's hypothesis cannot reproduce, even qualitatively, the experimental results.
The demonstration is easy : the fall of a secondary dropplet would be rapidly affected by the air drag force ; the radial velocity would decrease, while the vertical velocity would reach a constant value. Accordingly, the theoretical correlation between ring diameter and fall height can be easily computed.
A possible way of elucidation of the phenomenon was initiated through discussions with several physicists, specialists in fluid dynamics. From these discussions it is clear that the hypothesis of a drop fragmentation is difficult to support. The behavior of drops has indeed been widely studied because of its importance in fields as distant as the optimization of the operation of inkjet printers or of weather forecasting. It is known that during the formation of a drop by detachment at the end of a capillary, droplets of small size, called satellite drops, appear sometimes, but they are always formed behind the main drop, and remain on the fall axis (Eggers, 2005). Additionnally, during a free fall in a static atmosphere, when the drop diameter is less than 6 mm (which is the case for the drops falling from stalactites tips), the drop is stable and there is no possible breakup (Villermaux and Bossa, 2009).
Photo 4 - Foggy atmosphere in the “Galerie du Metro” in Tham En
( R. Huttler, 2008)
Since the hypothesis of falling drops rupture must be rejected, another explanation has to be proposed. 
Rather than searching for a mechanism that would require special properties for the drops, ceiling or floor, we looked for a link with the atmosphere properties. A common denominator is indeed the presence of mist in the galleries where the circles were observed (Fig. 4, in february, the heart of the dry season in Laos) and slow air circulation related to the large width passages.
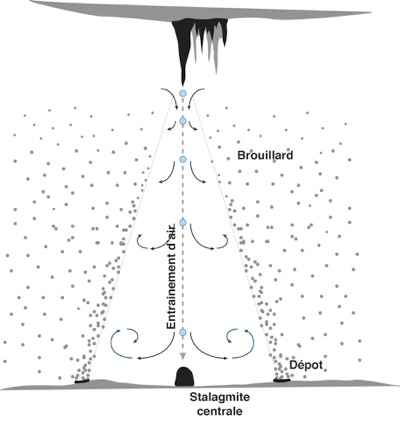
The mechanism could be : during their fall, the train of drops disturb the surrounding atmosphere, causing a downward widening flow which pushes the mist microdroplets away from the fall axis and eventually helps their accretion. The mist deposition would accordingly be located on a ring (Fig. 3). The frequency of falling drops could be the factor deciding why the rings appear only under some stalactites.
To support this hypothesis, we can notice that the rings are the footprint of a cone whose apex angle is equal to 18°. This value is substantially that of the angle of growth of a turbulent jet of air (20°, Sakiadis, 1984).
Another mean of validation, indirect, would be to search for a possible link between the formation mechanism and the chemical composition of the ring deposits. We performed a minimal sampling of the circle presented in photo 1. Samples taken in 2008 on the ring were completed in 2010 with small fragments of the stalagmite and intra- and extra-annular ground. X-ray spectroscopy using a scanning electron microscope was used to obtain the samples composition. Around the ring, sulfur (sulfate) is present in amounts sufficient to allow the formation of gypsum cristals, but is absent in the ring. Phosphorus (phosphate) is present in large amount in the ring, but is not found in the other samples.
This abnormal presence of phosphate must be connected to the presence of heaps (several hundred m2, over one meter thick) of swiftlet guano in the entrance gallery. Dust particles transported in the cave could serve as nucleation for mist droplets. Their deposition would explain the presence of large phosphate amounts in the ring. In the absence of dust, deposition of water droplets results in a local cleaning of the ground. The circles then appears as a clear ring on darker background.
Figure 3 - Mecanism proposed to explain the formation of cave rings.
Rares ? Not so much !
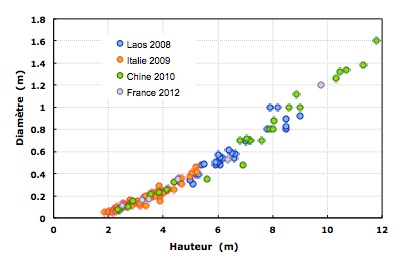
We met again the mysterious circles at the bottom of the Cave de Vitalis, a well known cave on the edge of the Causse du Larzac (in other words in our own garden !). The first one, on a steeply inclined flowstone, near the Turtle Gour, was very distorted. In contrast, the second one, found at the end of the Lake Gallery, was perfect, although less spectacular than its Lao counterparts. Some time after, we took advantage of a visit to the "secret" galleries of the Clamouse cave, not yet opened to tourists visits, to go cave-ring hunting. And we were not disappointed, with a dozen catches that nicely complement our collection of measurements. Another couple of circles was observed recently in the TM71, in the upper valley of the Aude river (south of France). In each case, the circles were formed in areas poorly ventilated, where a mist appears after periods of heavy rain.
Figure 4 - Correlation between ring diameter and ceilling height
Easy : the cave ring diameter (in meters) is always close to 0.14 x (height - 2)... Happy ring hunting ! Keep us informed !
Bibliographie
Auler A., 1993. Les cercles de calcite de la "Lappa do Bezerra" (Sao Domingos, Goyas, Brésil). Karstologia, 22, 2, 55-56.
Brouquisse F., Faverjon M., 2005. Rapport d'exploration 2002-2004. CREI. Fédération Française de Spéléologie.
Eggers J., 2005. Drop formation – an overview. ZAMM. Z. Angew. Math. Mech. 85, 6, 400-410.
Gunn R., Gilbert D., 1949. The terminal velocity of fall for water droplets in stagnant air. J. Meteorology, 6, 243-248
Hill C., Forti, P., 1998. Cave minerals of the world (2nd ed.), Huntsville, Alabama, NSS.
Hunt J.C.R, Delfos R., Eames I., Perkins R.J., 2007. Vortices, Complex Flows and Inertial Particles. Flow Turbulence Combust., 79, 207–234.
Montanaro L., 1992. Osservazioni sui ‘‘cerchi’’ della Grotta del Sorell. Boll. Gr. Speleol. Sassarese, 13, 21–22.
Nozzoli F., Bevilacqua S., Cavallari L., 2009. The genesis of cave rings explained using empirical and experimental data. J. Cave and Karst Studies, 71, 2, 130–135.
Renouard L., Gillet M., Lapie G., Scherk G., 2001. Spélaologie 2000 – Rapport d'expédition CREI. Fédération Française de Spéléologie.
Sakiadis B.C., 1984. Fluid and particle mechanics. in Perry's Chemical Engineer Handbook, Perry, Green, Maloney Edts, McGraw-Hill, Paris, p. 5-27.
Villermaux E., Bossa B., 2009. Single-drop fragmentation determines size distribution of raindrops. Nature Physics, 5, 697-702.